Introduction
The Neon Helium Productions (NeHe) online tutorials (http://nehe.gamedev.net/) are the best resources available for coders trying to learn specific OpenGL rendering techniques in C/C++. The CL-OpenGL library (http://common-lisp.net/project/cl-opengl/) is, to my mind, the most straightforward mapping of the OpenGL, GLU, and GLUT APIs into Common Lisp. In this series, I hope to combine the best of both so that the aspiring Lisp coder can quickly access these OpenGL techniques.
I am aware that someone else reworked the first six tutorials for CL-OpenGL. However, I can’t track those down any longer. The original website is gone. I am also aware that I won’t have a great deal of time for this sort of coding in the near future, but I hope to tackle a bunch of these this summer. Most of the tutorials are fairly short.
In this first tutorial, we’re going to generate a simple template file that opens an OpenGL window.
#<:use "glut-template.lisp">
Here is the resulting: intro.lisp.
Setting Up CL-OpenGL
To get started with CL-OpenGL, you will need a Lisp implementation that supports ASDF and CFFI, a git client, and OpenGL libraries. I will probably flesh this section out at some later date. For now, I am just barely going to touch on the prerequisites.
Lisp implementation.
CFFI.
git client.
OpenGL libraries. (Under Windows, you may need FreeGlut.dylib.)
Once you have all of the above, you will need to clone the CL-OpenGL repository so that you have the sources on your machine. The CL-OpenGL git repository is http://github.com/3b/cl-opengl.git.
% cd /where/you/want/to/put/the/cl-opengl/sources
% git clone http://github.com/3b/cl-opengl.git
You need to ensure that the cl-opengl/ directory that you just created is included in your asdf:*central-registry* list. For example, I have this in my ~/.sbclrc file
(dolist (subdir (list ;; ... some other packages ...
#P"cl-opengl/"))
(push (merge-pathnames subdir #P"/usr/local/asdf-install/site/")
asdf:*central-registry*))
Basic Template
Once you have CL-OpenGL installed, you’ll be able to use the following template.
#<:use "load opengl">
(defclass my-window (glut:window)
()
(:default-initargs :width 400 :height 300
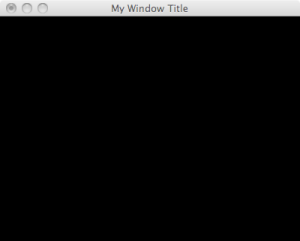
:title "My Window Title"
:x 100 :y 100
:mode '(:double :rgb :depth)))
#<:use "initialization method">
#<:use "additional glut methods">
#<:use "create an instance of our window">
Loading OpenGL
Usually, we’re going to load OpenGL, GLU, and GLUT.
(require :asdf) ; need ASDF to load other things
(asdf:load-system :cl-opengl) ; load OpenGL bindings
(asdf:load-system :cl-glu) ; load GLU bindings
(asdf:load-system :cl-glut) ; load GLUT bindings
Setting up OpenGL
Our initialization method can do anything we need to do in terms of loading textures or fonts or what-have-you. We could do some with the normal CLOS initialize-object method if it is stuff we can do before OpenGL is initialized. For our purposes though, we need to wait until after OpenGL is ready but before our window is displayed so we make sure to go before the glut:display-window call.
(defmethod glut:display-window :before ((win my-window))
#<:use "prepare opengl">
)
To prepare the default OpenGL environment that we’re going to use, we’re first going to turn on smooth shading. This allows colors to blend across our polygons. Later tutorials will go into more detail about smooth shading.
(gl:shade-model :smooth) ; enables smooth shading
The next line here sets the color used to clear the screen. OpenGL color values range from zero to one with zero being the darkest and one being the brightest. The parameters here are (in order) the red, green, blue, and alpha channels. The alpha channel doesn’t really come into play when clearing the screen, so it doesn’t much matter in this instance. We’re going to use a black background.
(gl:clear-color 0 0 0 0) ; background will be black
The next several lines prepare the depth buffer. OpenGL keeps a variety of buffers that are the same dimensions as your window. You were probably expecting the color buffer that stores the actual pixel values that are rendered on the screen. The depth can be used to keep track of the depth of the last item drawn to the screen or to prevent an object from drawing if it doesn’t have a depth thats less than the current value of the depth buffer for the current pixel.
(gl:clear-depth 1) ; clear buffer to maximum depth
(gl:enable :depth-test) ; enable depth testing
(gl:depth-func :lequal) ; okay to write pixel if its depth
; is less-than-or-equal to the
; depth currently written
We are also going to tell OpenGL that we’d like it to make things in perspective look as nice as possible.
; really nice perspective correction
(gl:hint :perspective-correction-hint :nicest)
Display function
Almost all applications will need at least a GLUT display function. Usually, they will do more than this, but this will get us started.
(defmethod glut:display ((win my-window))
(gl:clear :color-buffer-bit :depth-buffer-bit)
(gl:load-identity))
Resizing the window
The following method gets called when your window is first created and any time your window is resized. We will use it to prepare our projection matrix.
(defmethod glut:reshape ((win my-window) width height)
#<:use "glut reshape -- prepare viewport">
#<:use "glut reshape -- prepare projection">
#<:use "glut reshape -- switch to model view">
)
To initialize the viewport, we simply take the given width and height and use them as the horizontal and vertical extents of our coordinate system.
(gl:viewport 0 0 width height) ; reset the current viewport
Next, we’re going to prepare the projection matrix. We’re going to set things up for a perspective view so that distant objects appear smaller than closer objects. We’re going to assume that the window accounts for a 45-degree field of view from left to right. We’re going to assume that objects in our scene can be anywhere from 1/10 to 100 units in front of our viewpoint.
First, we switch into the mode where matrix commands will change the projection matrix. Then, we make sure we’re starting from the identity matrix. Then, we prepare our perspective transformation assuming 45-degrees from left-to-right and a proportional amount from top-to-bottom (taking care not to divide by zero in the proportion).
(gl:matrix-mode :projection) ; select the projection matrix
(gl:load-identity) ; reset the matrix
;; set perspective based on window aspect ratio
(glu:perspective 45 (/ width (max height 1)) 1/10 100)
Once we are done setting up the projection matrix, we need to switch back to the model-view matrix so that further transforms will affect the space we’re viewing rather than where we are viewing things from.
(gl:matrix-mode :modelview) ; select the modelview matrix
(gl:load-identity) ; reset the matrix
Creating and displaying our window
To create and display an instance of our window, we simply go ahead and create and instance and pass it to glut:display-window.
(glut:display-window (make-instance 'my-window))